Platonic solids inscribed into another Platonic solid using maximal configuration.
Computer generated models from Moritz Firsching's article about computing maximal side lengths of inscribed polytopes. *
After making Da Vinci's divine proportion polyhedra models, see blog posts https://papercraftetc.blogspot.com/2020/08/a-stem-project-constructing-da-vincis.html and https://papercraftetc.blogspot.com/2020/08/a-stem-project-constructing-da-vincis_21.html, I decided to inscribe the polyhedra models into one another. Pacioli wrote in his Divine Proportion book about these inscriptions.
The five Platonic solids, the tetrahedron, cube, octahedron, icosahedron and dodecahedron, can be derived from a single one, the dodecahedron, which, according to Pacioli, "sustains the existence of all the others and governs the manifold harmonies and interrelations among all five". Since the dodecahedron is the basis for all others, Pacioli claimed that it would be only mathematically possible with a specific proportion which he named the "Divine Proportion".
Pacioli wrote about inscriptions of the five Platonic solids solids using the sphere method of calculating the side lengths of the interior polyhedron. I inscribed the Platonic solids as I thought Da Vinci would have done if he had the Silhouette software and cutting technology.
I found out while making the models that as the inscribed polyhedron’s size approached a sphere, the size calculations became more complex and difficult to calculate. With some investigation on the internet, I discovered an article about maximizing the side lengths of the interior polyhedron. The computations for six of the polyhedra were just calculated in 2018 by the article's author, Moritz Firsching. These maximal size calculations were in the annals of unsolved geometric problems for many centuries. Using Firsching’s calculations, I was able to complete the 20 inscribed Platonic solid models.
"Computing maximal copies of polytopes contained in a polytope", by Moritz Firsching, Institut fu ̈r Mathematik FU Berlin Arnimallee 2 14195 Berlin Germany, July 16, 2018 https://arxiv.org/pdf/1407.0683.pdf *
The following calculations were cited in this paper:
Maximum side lengths of polyhedron inscribed in another polyhedron.
Using the above calculations, I created my models. I used Glue Dots to affix the inscribed polyhedra. I also used clear acetate to make a base for some of the models so that I could adhere the inscribed polyhedra model to something since the point of contact was sometimes in mid-space in the outer hollow polyhedron.
Here is the PDF. I used 65lb. cardstock to make the models.
Here is the .Studio file.
Here is the SVG.
Polyhedron Inscribed in a Tetrahedron
I made the side length of the Tetrahedron 3 inches in order to get a larger sized model.
1). Cube in a Tetrahedron. The side length of the cube was calculated as 0.296 x 3 = 0.888 inches.
0.888 in. side length Cube in a 3 in. side length Tetrahedron
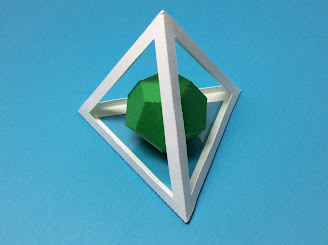
2). Octahedron in a Tetrahedron. The side length of the octahedron was calculated as 0.500 x 3 = 1.5 inches.
1.5 in. side length Octahedron in a 3 in. side length Tetrahedron
3). Dodecahedron in a Tetrahedron. The side length of the dodecahedron is calculated as 0.163 x 3 = 0.489 inches.
0.489 in. side length Dodecahedron in a 3 in. side length Tetrahedron
4). Icosahedron in a Tetrahedron. The side length of the icosahedron is calculated as 0.27 x 3 = 0.81 inches.
0.81 in. side length Icosahedron in a 3 in. side length Tetrahedron
Polyhedron Inscribed in a Cube
I made the side length of the Cube 2 inches in order to get a larger sized model.
5). Tetrahedron in a Cube. The side length of the tetrahedron is calculated as 1.414 x 2 = 2.828 inches.
2.828 in. side length Tetrahedron in a 2 in. side length Cube
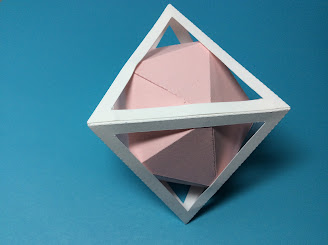
6). Octahedron in a Cube. The side length of the octahedron is calculated as 1.06 x 2 = 2.12 inches.
2.12 in. side length Octahedron in a 2 in. side length Cube
7). Dodecahedron in a Cube. The side length of the dodecahedron is calculated as 0.394 x 2 = 0.788 inches.
0.788 in. side length dodecahedron in a 2 in. side length Cube
8). Icosahedron in a Cube. The side length of the icosahedron is calculated as 0.618 x 2 = 1.236 inches.
1.236 in. side length icosahedron in a 2 in. side length Cube
Polyhedron Inscribed in an Octahedron
I made the side length of the Octahedron 3 inches in order to get a larger sized model.
9). Tetrahedron in an Octahedron. The side length of the tetrahedron is calculated as 1 x 3 = 3 inches.
3 in. side length Tetrahedron in a 3 in. side length Octahedron
10). Cube in an Octahedron. The side length of the cube is calculated as 0.586 x 3 = 1.758 inches.
1.758 in. side length cube in a 3 in. side length Octahedron
11). Dodecahedron in an Octahedron. The side length of the dodecahedron is calculated as 0.313 x 3 = 0.939 inches.
0.939 in. side length dodecahedron in a 3 in. side length Octahedron
12). Icosahedron in an Octahedron. The side length of the icosahedron is calculated as 0.54 x 3 = 1.62 inches.
1.62 in. side length icosahedron in a 3 in. side length Octahedron
Polyhedron Inscribed in a Dodecahedron
The side length of the Dodecahedron is 1 inch
13). Tetrahedron in a Dodecahedron.
2.288 in. side length Tetrahedron in a 1 in. side length Dodecahedron
14). Cube in a Dodecahedron.
1.618 in. side length cube in a 1 in. side length Dodecahedron
15). Octahedron in a Dodecahedron.
1.851 in. side length octahedron in a 1 in. side length Dodecahedron
16). Icosahedron in a Dodecahedron.
1.309 in. side length icosahedron in a 1 in. side length Dodecahedron
Polyhedron Inscribed in an Icosahedron
I made the side length of the Icosahedron 2 inches in order to get a larger sized model.
17). Tetrahedron in an Icosahedron. The side length of the tetrahedron is calculated as 1.347 x 2 = 2.694 inches.
2.694 in. side length Tetrahedron in a 2 in. side length Icosahedron
18). Cube in an Icosahedron. The side length of the cube is calculated as 0.939 x 2 = 1.878 inches.
1.878 in. side length cube in a 2 in. side length Icosahedron
19). Octahedron in an Icosahedron. The side length of the octahedron is calculated as 1.181 x 2 = 2.362 inches.
2.362 in. side length octahedron in a 2 in. side length Icosahedron
20). Dodecahedron in an Icosahedron. The side length of the dodecahedron is calculated as 0.58 x 2 = 1.16 inches.
1.16 in. side length Dodecahedron in a 2 in. side length Icosahedron
No comments:
Post a Comment