A STEM Project: Slice Forms of Common Quadric Surfaces Part #1

Hyperbolic Paraboloid Slice Forms with Two Orientations and Ellipsoid Slice Forms with Two Orientations 

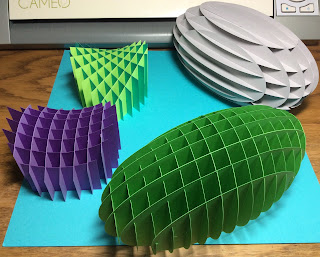
I made slice forms of six different quadric surfaces: Hyperbolic Paraboloid aka Monkey Saddle, Ellipsoid, Hyperboloids of One Sheet, Cone, Hyperboloids of Two Sheets and Elliptic Paraboloid.  This post is the first of three posts about quadric surface slice forms. This post will include the slice forms of the Hyperbolic Paraboloid and the Ellipsoid in two orientations because I think it is interesting to see this aspect for each figure.  The external structure is the same while the internal structure is different.

Quadric surfaces are the graphs of any equation that can be put into the general form

$$A{x}^2+B{y}^2+C{z}^2+Dxy+Exz+Fyz+Gx+Hy+Iz+J=0$$

where $A$, … , $J$ are constants. 

I made all of the slice forms by using this formula. I graphed the quadric surface with the Desmos online graphing calculator, https://www.desmos.com/calculator.  The image created represents the traces needed to form each slice. The slices were then divided by a constant interval of 0.5 inches to create the slits.

Here is the PDF.  I used a good quality 65 lb. cardstock.  The paper needs to be stiff and should not curl.  Otherwise the slices will curl. The slice forms are assembled by sliding the two center slices together.  Next, all of the upward facing slices are slid onto the center slices.  Lastly, all of the downward facing slices are slid onto the center slices.

https://drive.google.com/file/d/1EoaImjFM8zexugpC_xBbLuwbwHCFYacj/view?usp=sharing

Here is the .Studio file.

https://drive.google.com/file/d/1i5U3V0uhYatpJXvamilkpaoLdMlpRusY/view?usp=sharing

Here is the SVG. The SVG file goes beyond the viewable area.  To see the entire file, zoom out.

https://drive.google.com/file/d/1PXvJfShX7cjVCXGXuGKai4zANCZiY9qV/view?usp=sharing

Hyperbolic Paraboloid aka Monkey Saddle  These slice forms looks like a Pringle chip which is placed in a round canister.

Equation for a Hyperbolic Paraboloid.

$$\frac{x^2}{a^2}-\frac{y^2}{b^2}=\; z$$

Here is the graph of the purple colored Hyperbolic Paraboloid.

Side View of Hyperbolic Paraboloid Slice Forms with two Orientations

Notice that the external form of the slice forms are exactly the same.  They both have circular sides, flat  bottoms and a top surface which is curved.  It is interesting to note that the slices of the slice form on the left are all angled straight lines with the center slice parallel to its base. While the slice form slices on the right, has curved lines on top.

Top View of Hyperbolic Paraboloid Slice Forms in Two Orientations

Two Hyperbolic Paraboloid Slice Forms in Two Orientations

Lying Flat 

Please see this previous blog posting about how to assemble the Hyperbolic Paraboloid. https://papercraftetc.blogspot.com/2013/07/the-coolest-sliceform-hyperbolic.html

Ellipsoid

Graph of Ellipsoid showing both dimensions

Equation of an ellipsoid.

$$\mathrm{x2a2+y2b2+z2c2=1}$$

When a = b = c = 1, a sphere centered at the origin is formed. An ellipsoid is a “deformation” of the sphere such that the sphere gets either stretched or squeezed (depending on the values of a, b, c) in the x, y, z-directions.

Side View of Ellipsoid Slice Forms With Two Orientations

Please note: Both of the slice forms are the same length of 8 inches.  The size difference is an optical illusion due to the way I took the photo.

End view of Ellipsoid Slice Forms With Two Orientations

The orientation of the slices gives it a different internal structure while the external structure is exactly the same.

Two Ellipsoid Slice Form With Two Orientations

lying flat. 

When comparing a Hyperbolic Paraboloid  with the Elliptic Paraboloid, they both have the same features with the only difference is that the coefficients of the quadratic terms (x2,y2 ) have opposite signs.